Dividing radicals
In this article we explain how to solve divisions between radicals, whether they have equal or different indexes, with step-by-step exercises.
Table of Contents
Division of radicals with equal index
When two radicals have the same index, they are called homogeneous. The number that multiplies the root is called the coefficient. For example: *\sqrt{8}* and *4\sqrt{11}* are homogeneous radicals with coefficients *1* and *4* respectively.
To divide radicals with equal index, keep the index and combine the radicands inside the same root. Coefficients are divided outside the root.
*\dfrac{a\sqrt[n]{p}}{b\sqrt[n]{q}}=\dfrac{a}{b}\sqrt[n]{\dfrac{p}{q}}*
Division between roots can also be expressed as *\sqrt[n]{p}:\sqrt[n]{q},* but in our examples, we'll use fractional bars for convenience.
Example 1
*\dfrac{\sqrt{2}}{\sqrt{8}}*
Both radicals have coefficients of 1; to solve the division, integrate the radicands inside the same root.
*\dfrac{\sqrt{2}}{\sqrt{8}}=\sqrt{\dfrac{2}{8}}*
*=\sqrt{\dfrac{1}{4}}*
*=\dfrac{1}{2}*
It is advisable, if possible, to simplify the resulting root.
Example 2
*\dfrac{3\sqrt{40}}{4\sqrt{8}}*
Follow the same procedure as before, combining the radicals inside the same root and dividing the coefficients outside.
*\dfrac{3\sqrt{40}}{4\sqrt{8}}=\dfrac{3}{4}\sqrt{\dfrac{40}{8}}*
*=\dfrac{3}{4}\sqrt{5}*
Example 3
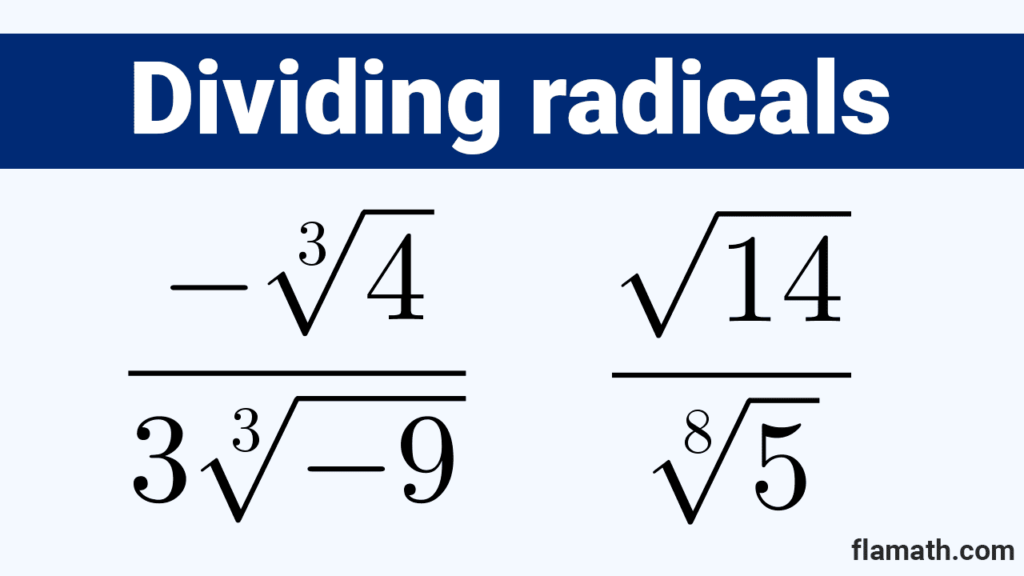
*\dfrac{-\sqrt[3]{4}}{3\sqrt[3]{-9}}*
Sign rules apply to both coefficients and radicands, and the procedure is the same as before.
*\dfrac{-\sqrt[3]{4}}{3\sqrt[3]{-9}}=-\dfrac{1}{3}\sqrt[3]{\dfrac{4}{-9}}*
*-\dfrac{1}{3}\sqrt[3]{-\dfrac{4}{9}}*
Example 4
If variables (letters) appear in the coefficients or radicands during division, follow the same rules as seen so far. Here are two cases:
*\dfrac{a\sqrt{6}}{b\sqrt{n}}=\dfrac{a}{b}\sqrt{\dfrac{6}{n}}*
*\dfrac{4\sqrt[5]{w}}{2\sqrt[5]{k}}=\dfrac{4}{2}\sqrt[5]{\dfrac{w}{k}}=2\sqrt[5]{\dfrac{w}{k}}*
Example 5
*\dfrac{\sqrt[3]{56}+\sqrt[3]{189}}{-5\sqrt[3]{7}}*
In this example, the numerator is a sum of roots. To solve the operation, you can first distribute the denominator among the terms and then proceed as usual in each case.
*\dfrac{\sqrt[3]{56}+\sqrt[3]{189}}{-5\sqrt[3]{7}}=\dfrac{\sqrt[3]{56}}{-5\sqrt[3]{7}}+\dfrac{\sqrt[3]{189}}{-5\sqrt[3]{7}}*
*=-\dfrac{1}{5}\sqrt[3]{\dfrac{56}{7}}-\dfrac{1}{5}\sqrt[3]{\dfrac{189}{7}}*
*=-\dfrac{1}{5}\sqrt[3]{8}-\dfrac{1}{5}\sqrt[3]{27}*
*=-\dfrac{1}{5}\cdot 2-\dfrac{1}{5}\cdot 3*
*=-1*
Practice exercises: solve the following divisions.
- *\dfrac{5\sqrt[5]{-243}}{\sqrt[5]{-81}}*
- *\dfrac{-7\sqrt{27}}{2\sqrt{12}}*
- *\dfrac{-4\sqrt{27}+5\sqrt{12}}{\sqrt{3}}*
- *\dfrac{6\sqrt{20}}{4\sqrt{5}}*
Solutions:
- *\dfrac{5\sqrt[5]{-243}}{\sqrt[5]{-81}}=5\sqrt[5]{\dfrac{-243}{-81}}=5\sqrt[5]{3}*
- *\dfrac{-7\sqrt{27}}{2\sqrt{12}}=-\dfrac{7}{2}\sqrt{\dfrac{27}{12}}=-\dfrac{7}{2}\sqrt{\dfrac{9}{4}}=-\dfrac{7}{2}\cdot \dfrac{3}{2}=-\dfrac{21}{4}*
- *\dfrac{-4\sqrt{27}+5\sqrt{12}}{\sqrt{3}}=\dfrac{-4\sqrt{27}}{\sqrt{3}}+\dfrac{5\sqrt{12}}{\sqrt{3}}=-4\sqrt{\dfrac{27}{3}}+5\sqrt{\dfrac{12}{3}}=-4\sqrt{9}+5\sqrt{4}=-4\cdot 3+5\cdot 2=-2*
- *\dfrac{6\sqrt{20}}{4\sqrt{5}}=\dfrac{6}{4}\sqrt{\dfrac{20}{5}}=\dfrac{3}{2}\sqrt{4}=\dfrac{3}{2}\cdot 2=3*
Division of radicals with different index
Two radicals with different indices are called heterogeneous. For example: *\sqrt[6]{3}* and *\sqrt[3]{5}* are heterogeneous radicals.
To divide radicals with different indices, it is first necessary to equalize them. Then, proceed with the process as seen before.
To equalize the indices, follow these steps:
- Find the least common multiple (LCM) of the indices.
- Divide the LCM by each index.
- Multiply the value obtained in the previous step by the original indices and the exponent of the corresponding radicand.
For a more detailed explanation of index equalization, refer to the article on multiplication of radicals. Read it for more information.
Example 1
*\dfrac{\sqrt{14}}{\sqrt[8]{5}}*
1. Find the LCM of the indices.
*LCM(2,8)=8*
2. Divide the LCM by each index.
First root: *\dfrac{8}{2}=4*
Second root: *\dfrac{8}{8}=1*
3. Multiply the values obtained by the indices and exponents of the corresponding radicand.
First root: *\sqrt{14}=\sqrt[2\cdot 4]{14^4}=\sqrt[8]{38416}*
Second root: *\sqrt[8]{5}=\sqrt[8\cdot 1]{5^1}=\sqrt[8]{5}*
Now that the indices are equal, we can proceed with the operation.
*\dfrac{\sqrt{14}}{\sqrt[8]{5}}=\dfrac{\sqrt[8]{38416}}{\sqrt[8]{5}}=\sqrt[8]{\dfrac{38416}{5}}*
Example 2
*\dfrac{4\sqrt[6]{2}}{5\sqrt[9]{2}}*
1. Find the LCM of the indices.
*LCM(6,9)=18*
2. Divide the LCM by each index.
First root: *\dfrac{18}{6}=3*
Second root: *\dfrac{18}{9}=2*
3. Multiply the values obtained by the indices and exponents of the corresponding radicand.
First root: *\sqrt[6]{2}=\sqrt[6\cdot 3]{2^3}=\sqrt[18]{8}*
Second root: *\sqrt[8]{2}=\sqrt[9\cdot 2]{2^2}=\sqrt[18]{4}*
Now we proceed to divide:
*\dfrac{4\sqrt[6]{2}}{5\sqrt[9]{2}}=\dfrac{4\sqrt[18]{8}}{5\sqrt[18]{4}}*
*=\dfrac{4}{5}\sqrt[18]{\dfrac{8}{4}}*
*=\dfrac{4}{5}\sqrt[18]{2}*
Practice exercises: solve the following divisions.
- *\dfrac{5\sqrt[3]{12}}{\sqrt{2}}*
- *\dfrac{4\sqrt{3}}{\sqrt[4]{2}}*
- *\dfrac{3\sqrt{10}-2\sqrt[3]{5}}{\sqrt{2}}*
- *\dfrac{2\sqrt[4]{5}}{\sqrt[3]{4}}*
Solutions:
- *\dfrac{5\sqrt[3]{12}}{\sqrt{2}}=\dfrac{5\sqrt[6]{12^2}}{\sqrt[6]{2^3}}=5\sqrt[6]{\dfrac{12^2}{2^3}}=5\sqrt[6]{18}*
- *\dfrac{4\sqrt{3}}{\sqrt[4]{2}}=\dfrac{4\sqrt[4]{3^2}}{\sqrt[4]{2}}=4\sqrt[4]{\dfrac{3^2}{2}}=4\sqrt[4]{\dfrac{9}{2}}*
- *\dfrac{3\sqrt{10}-2\sqrt[3]{5}}{\sqrt{2}}=\dfrac{3\sqrt{10}}{\sqrt{2}}-\dfrac{2\sqrt[3]{5}}{\sqrt{2}}=3\sqrt{\dfrac{10}{2}}-\dfrac{2\sqrt[6]{5^2}}{\sqrt[6]{2^3}}=3\sqrt{5}-2\sqrt[6]{\dfrac{25}{8}}*
- *\dfrac{2\sqrt[4]{5}}{\sqrt[3]{4}}=\dfrac{2\sqrt[12]{5^3}}{\sqrt[12]{4^4}}=2\sqrt[12]{\dfrac{125}{256}}*
FAQs
How to divide radicals?
To divide radicals, they must have the same index (be homogeneous). Otherwise, you must try to equalize the indices before operating.
How to divide radicals with equal index?
To divide radicals with equal index, the same index is maintained and the radicands are integrated and divided into the same root. The coefficients are divided outside.
How to divide radicals with different index?
To divide radicals of different index, you must first homogenize them (make them have the same index). Then, integrate the radicands into the same root and divide the coefficients outside.
Other articles that may interest you