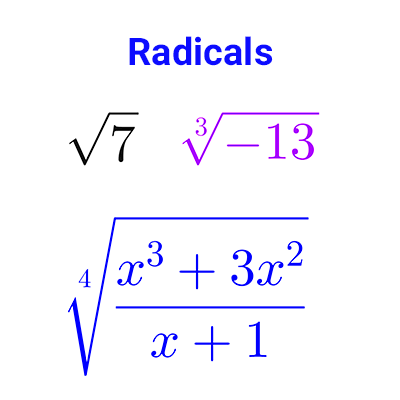
Radical operations
In this article we explain the basic operations with radical expressions: addition, subtraction, multiplication, division, potentiation and radication. We will see the rules for performing each one and examples. Also, we will see the processes of root simplification and rationalization. We work with roots of any index: square, cubic, etc.
Table of Contents
Addition and subtraction of radicals
To add or subtract radicals, they must be similar, meaning their roots must be identical. The procedure involves adding the coefficients and copying the same root.
*a\sqrt[n]{k}+b \sqrt[n]{k}=(a+b) \sqrt[n]{k}*
If the radicals are not similar, attempt to modify them to make them similar.
Examples:
*5\sqrt{5}+4\sqrt{5}=(5+4)\sqrt{5}=9\sqrt{5}*
*4\sqrt[3]{14}-10\sqrt[3]{14}=(4-10)\sqrt[3]{14}=-6\sqrt[3]{14}*
You can see more examples and what happens with non-similar radicals in this article:
Multiplication of radicals
To multiply two radicals, they must have the same index. The procedure involves multiplying the coefficients and the radicands separately, with the latter inside the same root with the same index.
*\sqrt[n]{a}\cdot \sqrt[n]{b}=\sqrt[n]{a\cdot b}*
*p\sqrt[n]{a}\cdot q\sqrt[n]{b}=p\cdot q\sqrt[n]{a\cdot b}*
If the radicals have different indices, it is necessary to equalize them by finding an equivalent radical.
Examples:
*-4\sqrt[3]{5}\cdot 6\sqrt[3]{4}=(-4\cdot 6)\sqrt[3]{5\cdot 4}=-24\sqrt[3]{20}*
*3\sqrt{5}\sqrt{2}\sqrt{2}=3\sqrt{5\cdot 2\cdot 2}=3\sqrt{20}*
Look at more examples and what to do with radicals of different indices in this article:
Division of radicals
To divide two radicals, they must have the same index. What is done is to keep the index and integrate the radicands into the same root. Coefficients are operated outside.
*\dfrac{a\sqrt[n]{p}}{b\sqrt[n]{q}}=\dfrac{a}{b}\sqrt[n]{\dfrac{p}{q}}*
If the radicals have different indices, it is necessary to equalize them first.
Examples:
*\dfrac{3\sqrt{48}}{4\sqrt{8}}=\dfrac{3}{4}\sqrt{\dfrac{48}{8}}=\dfrac{3}{4}\sqrt{6}*
*\dfrac{6\sqrt{20}}{4\sqrt{5}}=\dfrac{6}{4}\sqrt{\dfrac{20}{5}}=\dfrac{3}{2}\sqrt{4}=\dfrac{3}{2}\cdot 2=3*
You can see more examples and what to do with radicals of different indices in this article:
Combined operations
Mixed operations with radicals, also known as combined operations, are expressions containing additions, subtractions, multiplications, and divisions of roots. To solve them, it is important to know the procedures for each case.
Example:
*5\sqrt{3}\cdot(9\sqrt{2}+7\sqrt{18})=5\sqrt{3}\cdot(9\sqrt{2}+7\sqrt{3^2\cdot 2})=5\sqrt{3}\cdot(9\sqrt{2}+21\sqrt{2})*
*=5\sqrt{3}\cdot(30\sqrt{2})=5\cdot 30\sqrt{3}{\sqrt{2}}=150\sqrt{6}*
Radical exponentiation
Raising a root to an exponent is equivalent to raising the radicand to the power while maintaining the same index. This is possible due to a property of roots.
*(\sqrt[n]{a})^m=\sqrt[n]{a^m}*
*(\sqrt{2})^4=\sqrt{2^4}=\sqrt{16}=4*
*(\sqrt[3]{5})^2=\sqrt[3]{5^2}=\sqrt[3]{25}*
*(-\sqrt[5]{4})^6=\sqrt[5]{4^6}=\sqrt[5]{4096}*
Rooting of radicals
The root of a root is equal to a new root with the same radicand and an index equal to the product of the original indices. This equality arises from a property of roots.
*\sqrt[m]{\sqrt[n]{a}}=\sqrt[m\cdot n]{a}*
Examples:
*\sqrt[4]{\sqrt[6]{7}}=\sqrt[4\cdot 6]{7}=\sqrt[24]{7}*
*\sqrt{\sqrt[3]{5}}=\sqrt[2\cdot 3]{5}=\sqrt[6]{5}*
*\sqrt{\sqrt[5]{\sqrt{14}}}=\sqrt[2\cdot 5\cdot 2]{14}=\sqrt[20]{14}*
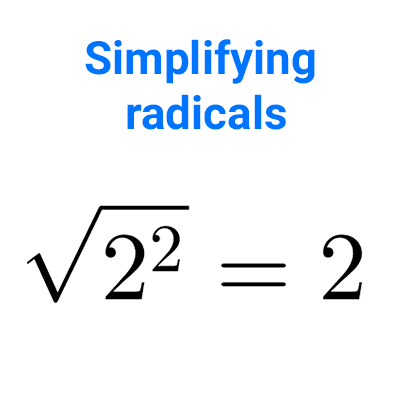
Simplification
Simplifying a radical involves writing it in the simplest form possible, so that it has the lowest index, no factors can be extracted from the radicand, and there are no fractions in it.
Examples:
*\sqrt[3]{7^3}=7*
*\sqrt[7]{20^{14}}=20^{\frac{14}{7}}=20^2=400*
*\sqrt[3]{2^{11}}=2^3\sqrt[3]{2^2}=8\sqrt[3]{4}*
See more examples and procedures in the following article:
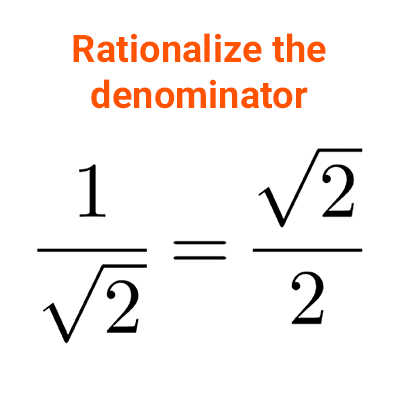
Rationalization
Rationalizing is rewriting a quotient so that radicals are removed from the numerator or denominator.
Examples:
*\dfrac{1}{\sqrt{2}}=\dfrac{1}{\sqrt{2}}\cdot \dfrac{\sqrt{2}}{\sqrt{2}}=\dfrac{\sqrt{2}}{(\sqrt{2})^2}=\dfrac{\sqrt{2}}{2}*
*\dfrac{\sqrt{8}}{\sqrt{3}}=\dfrac{\sqrt{8}}{\sqrt{3}}\cdot \dfrac{\sqrt{8}}{\sqrt{8}}=\dfrac{(\sqrt{8})^2}{\sqrt{3\cdot 8}}=\dfrac{8}{\sqrt{24}}*
*\dfrac{1}{\sqrt[3]{7}}=\dfrac{1}{\sqrt[3]{7}}\cdot \dfrac{\sqrt[3]{7^2}}{\sqrt[3]{7^2}}=\dfrac{\sqrt[3]{7^2}}{\sqrt[3]{7^3}}=\dfrac{\sqrt[3]{49}}{7}*
See more examples and cases of rationalization in the following article:
Other articles that may interest you